CALCULATION OF NARROWER CONFIDENCE INTERVALS FOR TREE MORTALITY RATES WHEN WE KNOW NOTHING BUT THE LOCATION OF THE DEATH/SURVIVAL EVENTS

October 15, 2019

The Science
Very few trees die. That results in very sparse information and great uncertainty about mortality. This work develops a technique to use spatial information to increase accuracy in the estimation of mortality.

The Impact
The general method described here, with modifications, could be applied to reduce uncertainty in the estimation of proportions related to any temporally or spatially structured phenomenon with two possible outcomes.

Summary
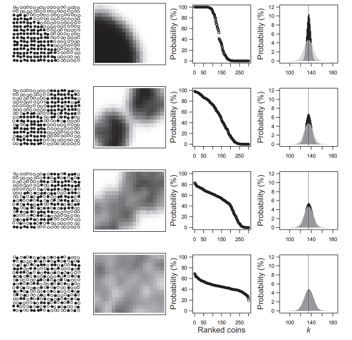
Many ecological applications, like the study of mortality rates, require the estimation of proportions and confidence intervals for them. The traditional way of doing this applies the binomial distribution, which describes the outcome of a series of Bernoulli trials. This distribution assumes that observations are independent and the probability of success is the same for all the individual observations. Both assumptions are obviously false in many cases. I show how to apply bootstrap and the Poisson binomial distribution (a generalization of the binomial distribution) to the estimation of proportions. Any information at the individual level would result in better (narrower) confidence intervals around the estimation of proportions. As a case study, I applied this method to the calculation of mortality rates in a forest plot of tropical trees in Lambir Hills National Park, Malaysia. I calculated central estimates and 95% confidence intervals for species‐level mortality rates for 1,007 tree species. I used a very simple model of spatial dependence in survival to estimate individual‐level risk of mortality. The results obtained by accounting for heterogeneity in individual‐level risk of mortality were comparable to those obtained with the binomial distribution in terms of central estimates, but the precision increased in virtually all cases, with an average reduction in the width of the confidence interval of ~20%. Spatial information allows the estimation of individual‐level probabilities of survival, and this increases the precision in the estimates of mortality rates. The general method described here, with modifications, could be applied to reduce uncertainty in the estimation of proportions related to any spatially structured phenomenon with two possible outcomes. More sophisticated approaches can yield better estimates of individual‐level mortality and thus narrower confidence intervals.

Figure. Experiment involving tossing 300 coins in a table. First column: the outcome (head = black, tail = white). It is clear that there are spatial processes at play. Second column: “probability of head” map. Third column: the 300 coins ranked by their probability of heads. Fourth column: the binomial distribution (gray bars) vs. the Poisson binomial (black bars). The Poisson binomial is more certain about the outcome when there is stronger spatial pattern.

Contacts (BER PM): Daniel Stover, SC-23.1, Daniel.Stover@science.doe.gov (301-903-0289)

PI Contact: Gabriel Arellano, University of Michigan, gabriel.arellano.torres@gmail.com

Funding
This study was developed as part of the Next Generation Ecosystem Experiments‐Tropics, funded by the U.S. Department of Energy (Office of Science, Office of Biological and Environmental Research). The Lambir 52‐ha plot was established as a collaboration between the Forest Department of Sarawak, Malaysia, Harvard University (NSF awards DEB‐9107247 and DEB‐9629601) and Osaka City University (grants 06041094, 08NP0901 and 09NP0901). The research has been supported by the Asia program of the Arnold Arboretum (Harvard University), the Center for Tropical Forest Science (Smithsonian Tropical Research Institute), the NSF award DEB‐1545761 to Stuart J. Davies, and the Sarawak Forest Department.

Publications
Arellano, G. (2019), Calculation of narrower confidence intervals for tree mortality rates when we know nothing but the location of the death/survival events, Ecology and Evolution, 9, 9644-9653. [DOI: https://doi.org/10.1002/ece3.5495]